The differential equation that is used, in the Black-Scholes model, to calculate the price or theoretical value of a European option on a non-dividend-paying stock. This equation is based on a neutral hedge established with risk-offsetting positions in the option and its underlying. The input parameters to the equation are: spot price of the underlying, strike price of the option, interest rate, time to expiration, and volatility of the underlying.
The Black-Scholes equation for the fair value of a European call option on a nondividend paying stock is:
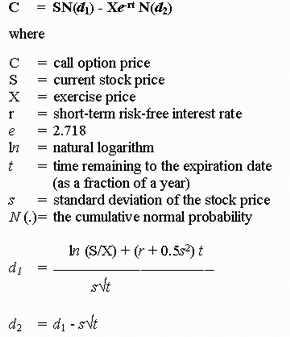
The simplicity of the Black-Scholes model and its consistency with the capital asset pricing model of portfolio theory contribute to its widespread popularity. However, it suffers from some fundamental shortcomings especially its assumption of static volatility and no-dividend payment on its underlying. As such, this model requires easing its narrow assumptions or the use of alternative versions in many real world applications.



Comments